Making Complexity
Simple

Paul Penfield, Jr. Professor of Electrical Engineering Department of Electrical Engineering    and Computer Science Massachusetts Institute of Technology Cambridge, MA 02139-4307 (617) 253-2506 penfield@mit.edu https://mtlsites.mit.edu/users/penfield/

Making Complexity Simple

Outline

Arcane

Pity

Perspective

Information

Course

Status

The Second Law of Thermodynamics

Surely one of science's most glorious accomplishments
Also one of the most profound and mysterious
What is this thing called "entropy?"
Does the Second Law really tell us which way clocks run?
Deep related concepts
Complexity
Randomness
Fluctuations
Dissipation
Order
The public's imagination is captured
Nobody really understands it
At least that is what we believed as graduate students
It is too complex to be taught

Why is entropy considered arcane?

Because people think it is part of thermodynamics
Thermodynamics really is hard to understand.
Many concepts -- heat, work, temperature, ...
You have to care about things like ideal gases.
Most engineers don't need to know thermodynamics.
Most don't care.

This is a shame

Entropy and the Second Law are taught as part of
   thermodynamics, so most people miss them.
... and thereby miss something pretty important
All scientists, all engineers, indeed all educated people need
   to understand that some operations are reversible and
   some are not.
They need to be able to tell them apart.
They need to know there is a way to quantify reversibility.
They need a "monotonic model"
To complement models of conserved quantities
Both are helpful for understanding the world
Both are prototypes for other quantities
Can we satisfy these very real needs somehow?
They have nothing to do with thermodynamics.
It's just that thermodynamics is where they have traditionally
   been taught.

Entropy is useful outside of thermodynamics

Thermodynamics always involves energy
Entropy need not
Outside of thermodynamics, without links to energy
Entropy is less complex
There are plenty of reversible and irreversible operations
The Second Law, or something much like it, exists
Monotonic models can be taught more easily
The more general the context, the simpler the concept
This is the secret of making complexity simple

War is too important to be left to the generals*

And entropy is too important to be left to the physicists

* George Clémenceau (1841 - 1929)
French Premier, 1906 - 1909, 1917 - 1920

Information is simpler and more general

Start with information
Entropy is one kind of information.
Entropy is information we do not have
See reversible and irreversible data transformations
In computation and communications
Note that irreversible operations destroy information
This is the Second Law in this context
Apply to a physical system with energy
Use maximum-entropy principle
Voila, thermodynamics!
Temperature is energy per bit of entropy (sort of)
Intensive vs. extensive variables
Second Law in traditional setting
Carnot efficiency
The basic idea of reversibility is not difficult to understand

We want to teach this stuff to freshmen

Why is this possible?
Today's students are different
Best to start from the known
Data, disks, Internet, packets, bits, ...
Consistent with the coming information age
Go toward the unknown
Thermodynamics, equilibrium, heat engines, refrigerators
Relevant to the current industrial age
Physical view of information . . . like energy, information
can be of many types
can be converted from one form to another
can exist in one place or another
can be sent from here to there
can be stored for later use
There are interesting applications
Biology (genetic code)
Communications
Quantum computing

Information and Entropy

A Freshman Course
12 weeks:
1. Bits
2. Codes
3. Compression
4. Errors
5. Probability
6. Communications
7. Processes
8. Inference
9. Entropy
10. Physical systems
11. Temperature
12. Myths
This course is NOT
Introduction to Computing
Introductory Communications
Thermodynamics 101

Course material

1. Bits
Restoring logic
Digital abstraction
Signals and streams
Boolean algebra
2. Codes
Bytes
Fixed-length codes
ASCII
Genetic code
Binary code, gray code
Variable-length codes
Morse code
Telegraph codebooks

Course material (cont)

3. Compression
Helps with low channel capacity
Codebooks
Irreversible -- fidelity requirement
JPEG
MP3
Reversible
Run length encoding
LZW
The LZW patent issue
4. Errors
Physical sources of noise
Detection -- parity
Correction
Triple redundancy
Hamming code

Course material (cont)

5. Probability
Racing odds
Random sources
coins, dice, cards
Probabilities are subjective
Information can be quantified
6. Communications
Model with source, coder, channel, decoder, receiver
Huffman codes
Symmetric binary channel
Lossless
Noisy
Client-server model
TCP and IP
Strategies for recovery from lost packets

Course material (cont)

7. Processes
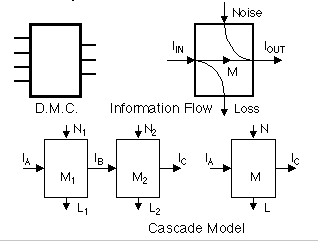
Discrete memoryless channel
Noise, loss
M = I_IN - L
= I_OUT - N
Cascade inequalities in L, N, and M
   L₁ <= L
L₁ + L₂ - N₁ <= L <= L₁ + L₂
8. Inference
Given received signal, what is input
How much information have we learned?

Course material (cont)

Discrete Memoryless Channel

Course material (cont)

9. Entropy
Entropy is information we do not have
Input probabilities consistent with constraints
Minimum assumptions, maximum entropy
Lagrange multipliers
Deer hunters
Fishermen
10. Physical systems
Energy per state
Expected value of energy
Boltzmann distribution
Lagrange multipliers are intensive variables
Equilibrium

Course material (cont)

11. Temperature
One of the Lagrange multipliers is temperature
Heat, work
Carnot efficiency
12. Myths
Order out of chaos
Miracle needed
Heat death
Evaporation of black holes
Difficulty of extensions to social science

Can freshmen actually learn this stuff?

We are finding out
Fall 1999, course development
Faculty: Paul Penfield, Seth Lloyd, Sherra Kerns
Students: small set of freshmen serving as guinea pigs
Spring 2000, pilot offering
Limited to 50 freshmen
Of course we have a Web site -- everybody does
https://mtlsites.mit.edu/users/penfield/6.095-s00/
Fall 2000, revisions, note writing
Spring 2001, first full offering
The devil is in the details
E.g., which is the best statistical mechanics model to use?

Some of the subtle points (risks)

Entropy is the information we don't have
Therefore entropy is subjective (some people don't like that)
Math generally simple -- discrete, not continuous processes
But Lagrange multipliers are not easy
Skill in modeling is still important
No magic here
At the freshman level you cannot go very deeply
All we can do is provide simple ideas to be built on later
We want to be consistent with later learning in specific areas
Stay in touch -- we will let you know how it turns out
If we are successful
The course will be permanent
We will help other universities start similar courses
We will advocate it as a science exposure in the liberal arts